1. Applegate, D., Bixby, R., Chvatal, V., Cook, W.: On the Solution of Traveling Salesman Problems. Documenta Mathematica (Journal der Deutschen Mathematiker-Verinigung, International Congress of Mathematicians), 645–656 (1998)Google Scholar
2. Ball, B.C., Webster, D.B.: Optimal Scheduling for Even-Numbered Team Athletic Conferences. AIIE Trans. 9, 161–169 (1977)Google Scholar
3. Barnhart, C., Johnson, E.L., Nemhauser, G.L., Savelsbergh, M.W.P., Vance, P.H.: Branch-And-Price: Column Generation for Huge Integer Programs. Oper. Res. 46, 316–329 (1998)zbMATHCrossRefMathSciNetGoogle Scholar
4. Benoist, T., Laburthe, F., Rottembourg, B.: Lagrange Relaxation and Constraint Programming Collaborative Schemes for Traveling Tournament Problems. In: CPAIOR, Wye College, pp. 15–26 (2001)Google Scholar
5. Easton, K.: Using Integer and Constraint Programming to Solve Sports Scheduling Problems. Doctoral Dissertation, Georgia Institute of Technology, Atlanta (2002) Google Scholar
6. Easton, K., Nemhauser, G.L., Trick, M.A.: The Traveling Tournament Problem: Description and Benchmarks, Principle and Practices of Constraint Programming. In: Walsh, T. (ed.) CP 2001. LNCS, vol. 2239, pp. 580–585. Springer, Heidelberg (2001)CrossRefGoogle Scholar
7. Gabow, H.N.: Data Structures for Weighted Matching and Nearest Common Ancestors with Linking. In: Proc. 1st Annu. ACM–SIAM Symp. Discr. Algorithms, pp. 434–443. SIAM, Philadelphia (1990)Google Scholar
8. Henz, M.: Scheduling a Major College Basketball Conference: Revisted. Oper. Res. 49, 163–168 (2001)zbMATHCrossRefMathSciNetGoogle Scholar
9. Kirkpatrick, D.G., Hell, P.: On the Complexity of a Generalized Matching Problem. In: Proc. 10th Annu. ACM Symp. Theory Computing. Association for Computing Machinery, New York, pp. 240–245 (1978)Google Scholar
10. Klabjan, D., Johnson, E.L., Nemhauser, G.L.: Solving Large Airline Crew Scheduling Problems: Random Pairing Generation and Strong Branching. Comput. Optim. Appl. 20, 73–91 (2001)zbMATHCrossRefMathSciNetGoogle Scholar
11. Russell, R.A., Leung, J.M.: Devising a Cost Effective Schedule for a Baseball League. Oper. Res. 42, 614–625 (1994)zbMATHCrossRefGoogle Scholar

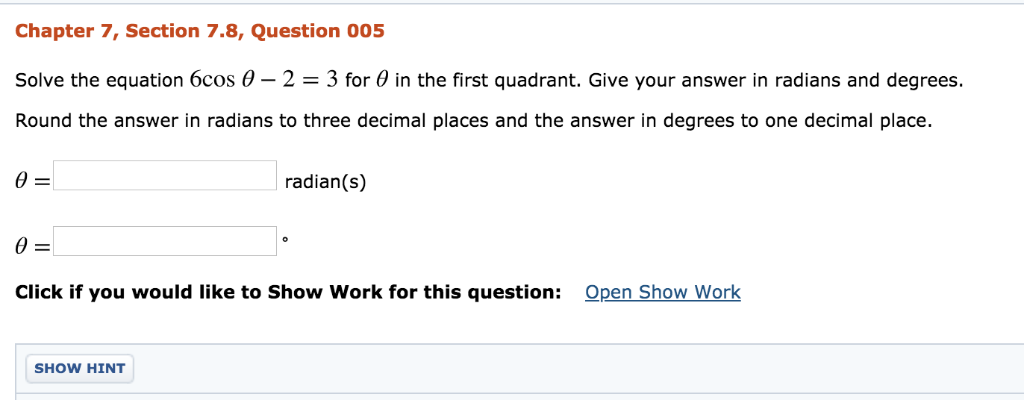
Math Problem Solving Questions

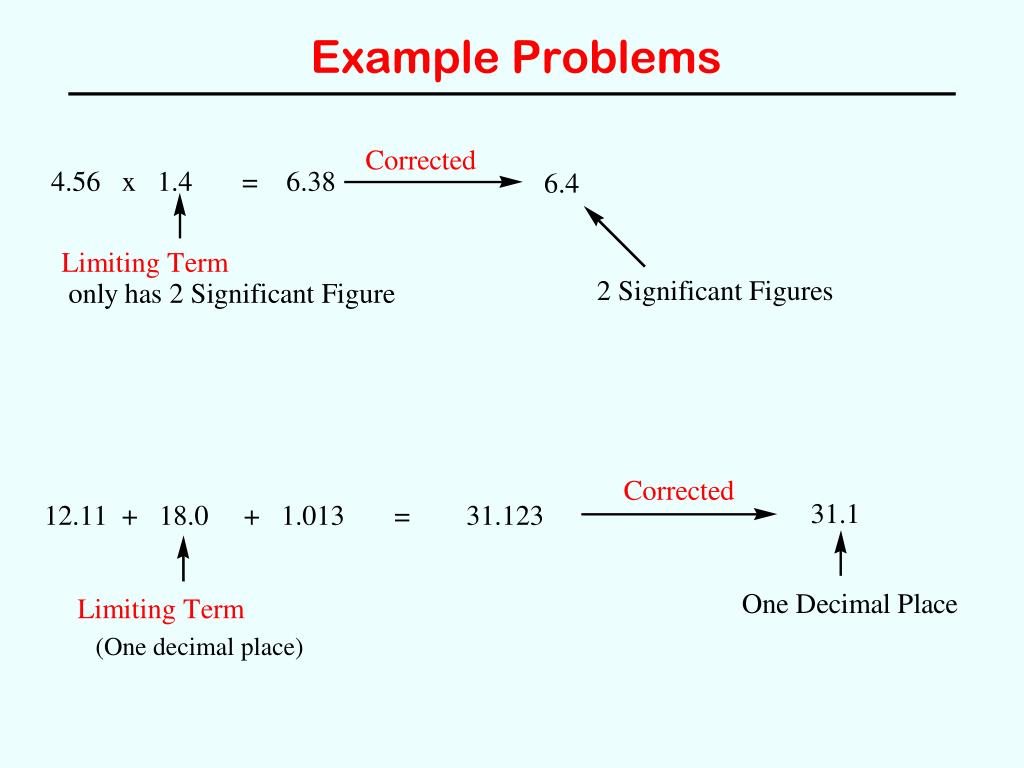
Solving Math Expressions

Problem-Solving Phase 1. Analysis and Specification. Understand (define) the problem and what the solution must do. General Solution (Algorithm). Specify the required data types and the logical sequences of steps that solve the problem. Follow the steps exactly to see if the solution really does solve the problem. Diverse group is the best solution for problem-solving tasks By Jared Wadley News Service. A diverse group of problem solvers is more likely to outperform a team of the best and brightest problem solvers, a U-M study shows. Individuals chosen from a diverse, randomly selected pool will offer different perspectives that could result in better.